Geometry Review Packet 1 Answer Key provides a comprehensive guide to the fundamental principles and practical applications of geometry. This meticulously crafted resource offers clear explanations, engaging examples, and insightful solutions to help students master the intricacies of geometric concepts and their real-world implications.
Delving into the foundational elements of geometry, this answer key elucidates the concepts of points, lines, planes, and angles, laying the groundwork for understanding more complex geometric structures. It further explores the measurement and properties of geometric figures, empowering students to determine lengths, areas, and volumes with precision.
Key Concepts in Geometry
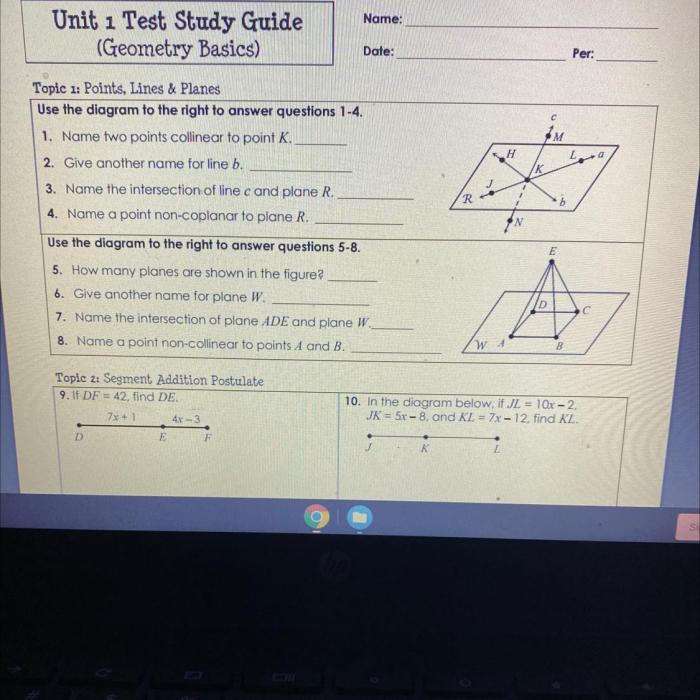
Geometry is the branch of mathematics that deals with the properties of shapes and figures. It is a fundamental subject in mathematics, with applications in various fields such as architecture, engineering, and design.
The basic building blocks of geometry are points, lines, planes, and angles. A point is a location in space that has no dimensions. A line is a one-dimensional object that extends infinitely in both directions. A plane is a two-dimensional object that extends infinitely in all directions.
An angle is formed by two rays that share a common endpoint.
Points
A point is the most basic element in geometry. It has no dimensions and is represented by a single letter, such as A, B, or C. Points can be located anywhere in space.
Lines
A line is a one-dimensional object that extends infinitely in both directions. It is represented by two points, such as AB or CD. Lines can be straight or curved.
Planes
A plane is a two-dimensional object that extends infinitely in all directions. It is represented by three points, such as ABC or DEF. Planes can be flat or curved.
Angles
An angle is formed by two rays that share a common endpoint. It is measured in degrees. Angles can be acute (less than 90 degrees), right (90 degrees), obtuse (greater than 90 degrees), or straight (180 degrees).
Measurement and Properties of Geometric Figures
Geometry is a branch of mathematics that deals with the measurement and properties of geometric figures. Geometric figures are two-dimensional or three-dimensional shapes that have specific properties and characteristics.
In this section, we will discuss the methods for measuring lengths, areas, and volumes of geometric figures. We will also explain the properties and characteristics of different geometric shapes, such as triangles, circles, and cubes.
Measuring Geometric Figures
The measurement of geometric figures is an important part of geometry. There are a number of different ways to measure geometric figures, depending on the type of figure and the information that is needed.
- Lengthis a one-dimensional measurement that describes the distance between two points.
- Areais a two-dimensional measurement that describes the amount of surface area of a figure.
- Volumeis a three-dimensional measurement that describes the amount of space that a figure occupies.
There are a number of different formulas that can be used to measure the length, area, and volume of geometric figures. These formulas are based on the properties of the figures and the relationships between their different dimensions.
Properties of Geometric Figures
Geometric figures have a number of different properties and characteristics. These properties can be used to identify and classify different types of figures.
- Shapeis the overall form of a figure.
- Sizeis the relative dimensions of a figure.
- Orientationis the position of a figure in space.
- Symmetryis the balance of a figure around a central point or axis.
The properties of geometric figures can be used to solve a variety of problems. For example, the properties of triangles can be used to find the missing side length or angle measure of a triangle.
Geometric Transformations

Geometric transformations are operations that change the position, size, or shape of a geometric figure. They are used in various fields, including mathematics, physics, computer graphics, and architecture. There are three main types of geometric transformations: translations, rotations, and reflections.
Translations
A translation moves a figure from one point to another without changing its size or shape. It is defined by a vector, which specifies the direction and distance of the movement. For example, translating a square 5 units to the right would move it 5 units in the positive x-direction.
Rotations
A rotation turns a figure around a fixed point called the center of rotation. It is defined by the angle of rotation and the direction of rotation. For example, rotating a triangle 90 degrees clockwise around its centroid would turn it 90 degrees in the clockwise direction.
Reflections
A reflection flips a figure over a line called the line of reflection. It is defined by the line of reflection and the direction of reflection. For example, reflecting a circle over the x-axis would flip it over the x-axis and create a mirror image of the original circle.
Coordinate Geometry
Coordinate geometry is a branch of mathematics that deals with the use of coordinates to represent points in a plane or space. The Cartesian coordinate system is the most commonly used coordinate system in coordinate geometry. It is a two-dimensional coordinate system that uses two perpendicular axes, the x-axis and the y-axis, to represent points in a plane.
To plot a point in the Cartesian coordinate system, we first find the x-coordinate of the point, which is the distance from the point to the y-axis. We then find the y-coordinate of the point, which is the distance from the point to the x-axis.
The coordinates of a point are written as an ordered pair (x, y), where x is the x-coordinate and y is the y-coordinate.
Plotting Points in the Coordinate Plane
- To plot a point in the coordinate plane, we first find the x-coordinate of the point, which is the distance from the point to the y-axis.
- We then find the y-coordinate of the point, which is the distance from the point to the x-axis.
- The coordinates of a point are written as an ordered pair (x, y), where x is the x-coordinate and y is the y-coordinate.
Graphing Equations in the Coordinate Plane
We can also use the Cartesian coordinate system to graph equations. To graph an equation, we first find the x- and y-intercepts of the equation. The x-intercept is the point where the graph of the equation crosses the x-axis, and the y-intercept is the point where the graph of the equation crosses the y-axis.
Once we have found the x- and y-intercepts, we can plot them on the coordinate plane and draw a line through the points. The line will be the graph of the equation.
Applications of Geometry in Real-World Contexts: Geometry Review Packet 1 Answer Key
Geometry finds extensive applications in numerous fields, shaping our built environment and aiding in scientific discoveries. From architectural marvels to engineering feats, geometric principles underpin the design and problem-solving processes.
Architecture
- Architects utilize geometry to create aesthetically pleasing and structurally sound buildings. Symmetry, proportion, and geometric shapes contribute to the visual appeal and stability of structures.
- For instance, the Parthenon in Greece exemplifies the harmonious use of geometric ratios and proportions, embodying the ideals of classical architecture.
Engineering, Geometry review packet 1 answer key
- Geometry plays a crucial role in engineering disciplines, enabling the design and analysis of complex structures. Bridges, skyscrapers, and aircraft are all engineered using geometric principles.
- In civil engineering, geometry is essential for calculating forces, stresses, and stability in structures, ensuring their safety and durability.
Art
- Geometry has a profound impact on the visual arts, influencing composition, perspective, and the creation of illusions.
- Artists employ geometric shapes, patterns, and symmetry to convey emotions, create visual harmony, and evoke a sense of order or chaos.
FAQ Explained
What is the purpose of Geometry Review Packet 1 Answer Key?
Geometry Review Packet 1 Answer Key provides comprehensive solutions and explanations to reinforce understanding of geometric concepts and their applications.
What topics are covered in this answer key?
Key concepts in geometry, measurement and properties of geometric figures, geometric transformations, coordinate geometry, and applications of geometry in real-world contexts.
How can this answer key help me improve my geometry skills?
By providing clear explanations, worked-out solutions, and practice exercises, this answer key helps students solidify their understanding of geometric principles and develop problem-solving abilities.